Cancer is a complex disease and thus is complicated to model. However, simple models that describe the main processes involved in tumoral dynamics, e.g., competition and mutation, can give us clues about cancer behavior, at least qualitatively, also allowing us to make predictions.

Scientists have identified a transcritical bifurcation at increasing mutation rates of the tumor cells. Such a bifurcation involves a scenario with dominance of healthy cells and impairment of tumor populations. Therefore, they have descrived that a slight increase beyond the critical mutation rate may be enough to have a fast response towards the desired state (i.e., low tumor populations) by applying directed mutagenic therapies.

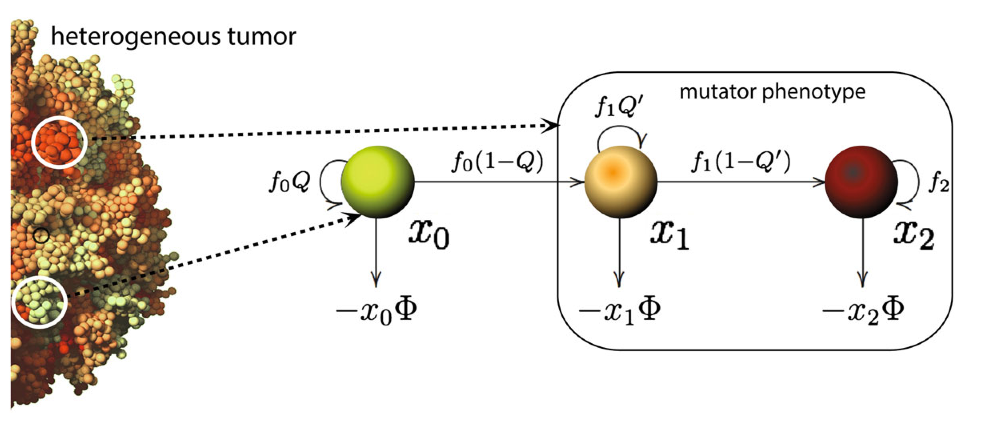
Reference Article: Castillo V, Lázaro JT, and Sardanyés J (2015). Dynamics and bifurcations in a simple quasispecies model of tumorigenesis. Computational & Applied Mathematics